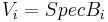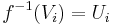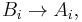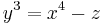Finite morphism

In algebraic geometry, a branch of mathematics, a morphism 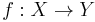$f: X \rightarrow Y$ of schemes is a finite morphism, if 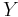$Y$ has an open cover by affine schemes

$V_i = Spec B_i$

such that for each 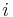$i$ ,

$f^{-1}(V_i) = U_i$

is an open affine subscheme 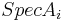$Spec A_i$ , and the restriction of f to 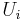$U_i$ , which induces a map of rings

$B_i \rightarrow A_i,$

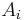makes $A_i$ a finitely generated module over $B_i$ .

1 Morphisms of finite type
2 Properties of finite morphisms
3 See also
4 References

Morphisms of finite type

There is another finiteness condition on morphisms of schemes, morphisms of finite type, which is much weaker than being finite.

Morally, a morphism of finite type corresponds to a set of polynomial equations with finitely many variables. For example, the algebraic equation

$y^3 = x^4 - z$

corresponds to the map of (affine) schemes $\mbox{Spec} \; \mathbb Z [x, y, z] / \langle y^3-x^4%2Bz \rangle \rightarrow \mbox{Spec} \; \mathbb Z$ or equivalently to the inclusion of rings $\mathbb Z \rightarrow \mathbb Z [x, y, z] / \langle y^3-x^4%2Bz \rangle$ . This is an example of a morphism of finite type.

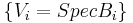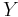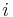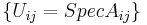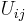The technical definition is as follows: let $\{V_i = Spec B_i\}$ be an open cover of $Y$ by affine schemes, and for each $i$ let $\{U_{ij} = Spec A_{ij}\}$ be an open cover of $f^{-1}(V_i)$ by affine schemes. The restriction of f to $U_{ij}$ induces a morphism of rings 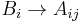$B_i \rightarrow A_{ij}$ . The morphism f is called locally of finite type, if 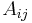$A_{ij}$ is a finitely generated algebra over $B_i$ (via the above map of rings). 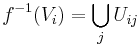If in addition the open cover $f^{-1}(V_i) = \bigcup_j U_{ij}$ can be chosen to be finite, then f is called of finite type.

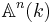For example, if $k$ is a field, the scheme $\mathbb{A}^n(k)$ has a natural morphism to 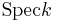${\mathrm{Spec}}k$ induced by the inclusion of rings $k \to k[X_1,\ldots,X_n].$ This is a morphism of finite type, but if $n>0$ then it is not a finite morphism.

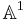On the other hand, if we take the affine scheme ${\mbox{Spec}} \; k[X,Y]/ \langle Y^2-X^3-X \rangle$ , it has a natural morphism to $\mathbb{A}^1$ given by the ring homomorphism $k[X]\to k[X,Y]/ \langle Y^2-X^3-X \rangle.$ Then this morphism is a finite morphism.

Properties of finite morphisms

In the following, f : X → Y denotes a finite morphism.

The composition of two finite maps is finite.
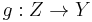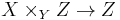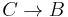Any base change of a finite morphism is finite, i.e. if $g: Z \rightarrow Y$ is another (arbitrary) morphism, then the canonical morphism $X \times_Y Z \rightarrow Z$ is finite. This corresponds to the following algebraic statement: if A is a finitely generated B-module, then the tensor product $A \otimes_B C$ is a finitely generated C-module, where $C \rightarrow B$ is any map. 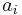The generators are $a_i \otimes 1$ , where $a_i$ are the generators of A as a B-module.
Closed immersion are finite, as they are locally given by 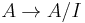$A \rightarrow A / I$ , where I is the ideal corresponding to the closed subscheme.
Finite morphisms are closed, hence (because of their stability under base change) proper. Indeed, replacing Y by the closure of f(X), one can assume that f is dominant. Further, one can assume that Y=Spec B is affine, hence so is X=Spec A. Then the morphism corresponds to an integral extension of rings B ⊂ A. Then the statement is a reformulation of the going up theorem of Cohen-Seidenberg.
Finite morphisms have finite fibres (i.e. they are quasi-finite). This follows from the fact that any finite k-algebra, for any field k is an Artinian ring. Slightly more generally, for a finite surjective morphism f, one has dim X=dim Y.
Conversely, proper, quasi-finite maps are finite. This is a consequence of the Stein factorization.
Finite morphisms are both projective and affine.

References

Hartshorne, Robin (1977), Algebraic Geometry, Graduate Texts in Mathematics, 52, New York: Springer-Verlag, ISBN 978-0-387-90244-9, MR 0463157

Finite morphism

Contents

Morphisms of finite type

Properties of finite morphisms

See also

References